A Drawer Contains 4 Pairs Of Blue Socks
A Drawer Contains 4 Pairs Of Blue Socks - Web a drawer contains 4 different pairs of socks. Two socks are chosen at random without replacement. The pairs have been separated out and you must take out a pair of socks. B) if 3 are drawn what is the probability of a match? Web a drawer contains 6 blue socks and 4 green socks. 4 are brown and 4 are blue. Therefore, favorable number of cases is 5 c 2 + 4 c 2. Number of pairs of gray socks = 3. I solved (a) by saying the number of different selections of socks is (82) = 28 ( 8 2) = 28 number of different matching combinations = 4 Web (10 points) a drawer contains four blue socks and six red socks. P (rr)=4/6xx3/5=12/30 if you picked a blue sock 2/6, if you picked another one it. There are 6 socks left, and 4 of them are not a match. We must pick a sock that is not a match to the first three. Web (10 points) a drawer contains four blue socks and six red socks. What is the minimum number. In how many ways can he do so?\n\\ ( \\begin {array} { l l l l } { \\text { (a) } 245 } & { \\text { (b) } 120 } & { \\text { (c) } 495 } & { \\text { (d) } 60. It feeds on fur, flannel, wool, soiled fabrics, and hair. Calculate the probability. I solved (a) by saying the number of different selections of socks is (82) = 28 ( 8 2) = 28 number of different matching combinations = 4 Three socks are randomly selected and removed in sequence. Web statistics and probability questions and answers. He has\nto select 4 socks from this set. Since we need to pick two socks, we. Whether you’re looking for a traditionally styled bedroom dresser or modernly designed chest of drawers, ikea’s collection of affordable dressers features a host of options to perfectly match your space. Therefore, favorable number of cases is 5 c 2 + 4 c 2. If you randomly pick two socks, what is the probability that you obtain a matching pair? Probability. B) if 3 are drawn what is the probability of a match? I solved (a) by saying the number of different selections of socks is (82) = 28 ( 8 2) = 28 number of different matching combinations = 4 Sample sizes are often small. It feeds on fur, flannel, wool, soiled fabrics, and hair. If six socks are taken. Web solution verified by toppr correct option is a. If a brown sock is pulled out and not replaced: 4 white, 3 blue, and 5 grey. Hence, the required probability is 5 c 2 + 4 c 2 9 c 2 = 4 9 Two socks are chosen at random without replacement. The question is asking for the probability of randomly choosing a pair of white socks from the given drawer of socks. What is the probability they both:a)match in colorb)do not match in color. 4 are brown and 4 are blue. We must pick a sock that is not a match to the first two. He has\nto select 4 socks from. Total pairs of socks= 4 + 5 + 3 = 12. If six socks are taken at random without replacement, compute the probability that there is at least one matching pair among these six socks. Web click here👆to get an answer to your question ️ \39. We must pick a sock that is not a match to the first two.. Web a drawer contains 4 different pairs of socks. What is the probability they both:a)match in colorb)do not match in color. If you randomly pick two socks, what is the probability that you obtain a matching pair? A drawer contains eight different pairs of socks. There are 8 socks left in the drawer. A drawer contains red, green, blue, and white socks with at least 2 of each color. We must pick a sock that is not a match to the first two. It feeds on fur, flannel, wool, soiled fabrics, and hair. There are 5 blue socks, 4 red socks and 3 green socks in debu's wardrobe. We must pick a sock. Therefore, the total number of cases is 9 c 2. There are 8 socks left in the drawer. B) if 3 are drawn what is the probability of a match? 4/9 out of 9 socks, 2 can be drawn in 9 c 2 ways. You have been provided with 20 pairs of socks within a box consisting of 4 red pairs, 4 yellow pairs, 4 green pairs, 4 blue pairs and 4 purple plairs. The probability of pulling out a brown sock at this point is 5 9, and the probability of pulling out a blue one is 4 9. Two socks are chosen at random without replacement. Since we need to pick two socks, we can find the total number of all possible pairs of socks using combinations. We must pick a sock that is not a match to the first three. So the probability of not picking a matching sock is $\frac {4} {6} = \frac {2} {3}$. There are a total of 12 socks in the drawer: Web a drawer contains four pairs of socks, with each pair a different color. (socks are not returned to the drawer once removed.) (i) assume that the first sock selected of the three is blue. 4 white, 3 blue, and 5 grey. There are 5 blue socks, 4 red socks and 3 green socks in debu's wardrobe. Titled this problem has been solved!
15 Clever Sock Storage Ideas You'll Want to Try Sponge Hacks
Solved A drawer contains 2 blue, 4 red, and 2 yellow socks.

Drawer in the Closet for Storing Underwear, Socks and Bras. Vertical

Pin by Yogita Salaskar on clothes to make Socks organizer, Diy sock

How to Organize Your Sock Drawer Martha Stewart YouTube
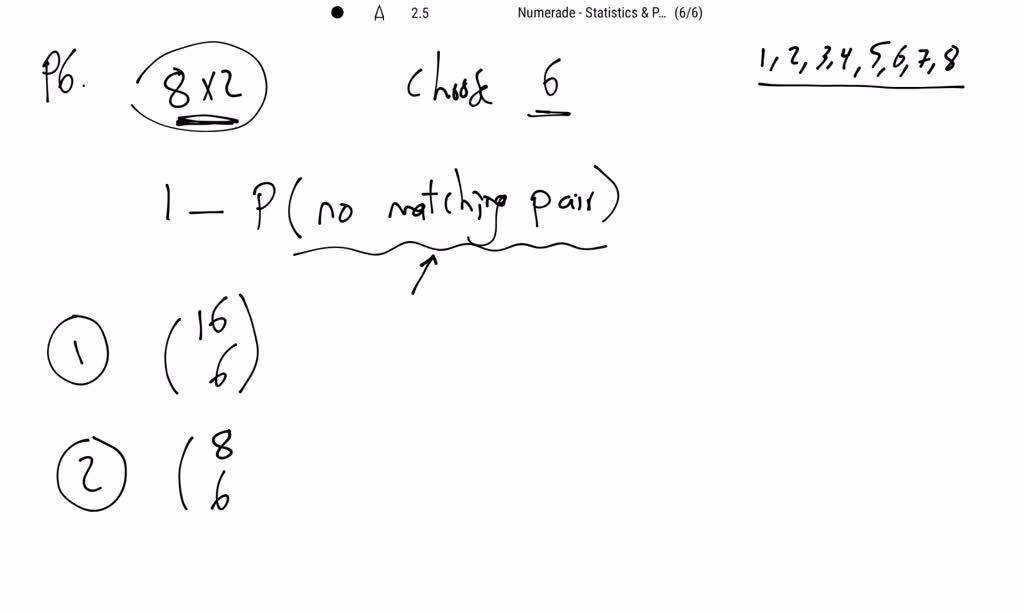
SOLVEDA drawer contains eight different pairs of socks. If six socks

SOLUTION A drawer contains 4 red socks, 6 white socks, and 10 blue
How Many Socks Make a Pair? A Mathematics Problem Owlcation
PJKnits I Gotta Sock Drawer!
MS. Simplicity Organize Your Socks And Underwear
But I Do Not Know How To Calculate The Probability That The First Two Socks Are Blue.
In How Many Ways Can He Do So?\N\\ ( \\Begin {Array} { L L L L } { \\Text { (A) } 245 } & { \\Text { (B) } 120 } & { \\Text { (C) } 495 } & { \\Text { (D) } 60.
We Must Pick A Sock That Is Not A Match To The First Two.
Web A Drawer Contains 4 Different Pairs Of Socks.
Related Post:
