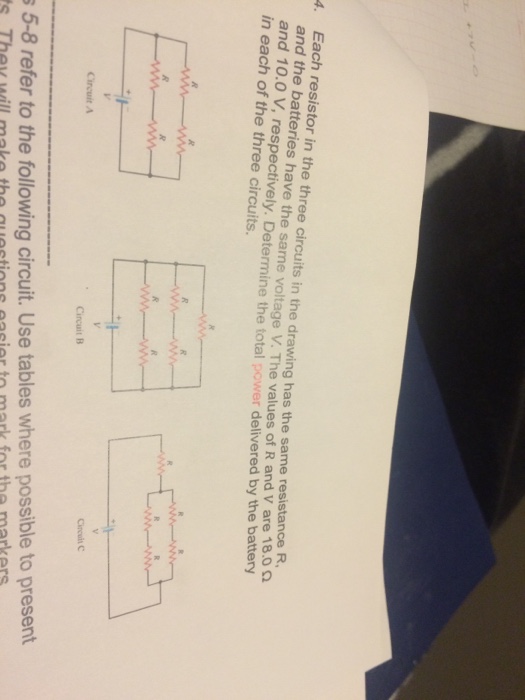
Each Resistor In The Three Circuits In The Drawing
Each Resistor In The Three Circuits In The Drawing - And that adds up to 300 ohms. Web each resistor in the three circuits in the drawing has the same resistance r, and the batteries have the same voltage v. We start by simplifying the parallel resistors r 2 and r 3. Continue, moving left until a single equivalent resistor represents the. Choose the direction of current flow. Determine the total power delivered by the battery in each of the three circuits. The values for r and v are 5 q and 6.0 v; Web one way to figure this out and to simplify the circuit is to replace all three of those resistors with a series resistor, rs, and that is, as we said here, is the sum, so it's 100, plus 50, plus 150. Web there are three primary resistors in series connection, resistors in parallel connection, and combinations of resistors in series and parallel connection. Web in the combination circuit sketched below, find the equivalent resistance for the circuit, find the total current through the circuit, and find the current through each individual resistor. Web each resistor in the three circuits in the drawing has the same resistance r, and the batteries have the same voltage v. Begin as far away as possible from the circuit location in question. Each resistor in the three circuits in the drawing has the same resistance r, and the batteries have the same voltage v. Web each resistor. Web there are three primary resistors in series connection, resistors in parallel connection, and combinations of resistors in series and parallel connection. R 3 = 10.0 ω. Determine the total power delivered by the battery in each of the three circuits. Web the equivalent resistance of a set of resistors in a series connection is equal to the algebraic sum. (b) the original circuit is reduced to an equivalent resistance and a voltage source. Teacher support emphasize that the voltage across each parallel resistor is the same, whereas the current may differ; (b) repeat part (a) for the circuit on the right. 1/r 23 =(1/180 ω)+(1/220 ω)=199 ω. Choose the direction of current flow. The battery has a voltage of v = 21 v, and the resistors have resistances of r1 = 50.0 ω, r2 = 25.0 ω and r3 = 10.0 ω. Each resistor has a value of 10.0 ohm. Determine the equivalent resistance between the points a and b, b and c, and a and c. Each resistor in the three circuits. Begin as far away as possible from the circuit location in question. (b) repeat part (a) for the circuit on the right. The current through each resistor; The values for r and v are 5 q and 6.0 v; Determine the total power delivered by the battery in. Determine the total power delivered by the battery in each of the three circuits. Choose the direction of current flow. Where n counts the element voltages around the loop. Web the drawing shows three different resistors in two different circuits. Web one way to figure this out and to simplify the circuit is to replace all three of those resistors. The battery has a voltage of v = 24.0 v, and the resistors have values of r 1 = 50.0 ω, r 2 = 25.0 ω, and. Determine the equivalent resistance between the points a and b, b and c, and a and c. (a) for the circuit on the left, determine the current through and the voltage across each. The current through each resistor; The sum of voltages around a loop is zero. Web strategy for simplifying a resistor network. You can also state kirchhoff's voltage law another way: The voltage drop across each resistor; Web there are three primary resistors in series connection, resistors in parallel connection, and combinations of resistors in series and parallel connection. Each resistor in the three circuits in the drawing has the same resistance r, and the batteries have the same voltage v. Web each resistor in the three circuits in the drawing has the same resistance r, and. And that adds up to 300 ohms. This circuit can be analyzed using kirchhoff’s rules. Replace series or parallel resistors with their equivalent resistor. R 3 = 10.0 ω. The battery has a voltage of v = 21 v, and the resistors have resistances of r1 = 50.0 ω, r2 = 25.0 ω and r3 = 10.0 ω. Determine the total power delivered by. Where n counts the element voltages around the loop. (a) three resistors connected in series to a voltage source. Web the right circuit diagram shows an equivalent resistance that replaces the three parallel resistors. This circuit consists of three resistors and two batteries connected in series. 1/r 23 =(1/180 ω)+(1/220 ω)=199 ω. Determine the total power delivered by the battery in. Determine the total power delivered by the battery in each of the three circuits. Web each resistor in the three circuits in the drawing has the same resistance r, and the batteries have the same voltage v. This circuit can be analyzed using kirchhoff’s rules. There is only one loop and no nodes. Let's say this is a circuit we have and this is a circuit we need to find out what's going on in the problem. The current from the power supply; Each resistor in the three circuits in the drawing has the same resistance r, and the batteries have the same voltage v. The values for r and v are 7 ω and 6.0 v, respectively. The battery has a voltage of v = 24.0 v, and the resistors have values of r 1 = 50.0 ω, r 2 = 25.0 ω, and.
Resistor Circuits The Electronics Hobby Blog
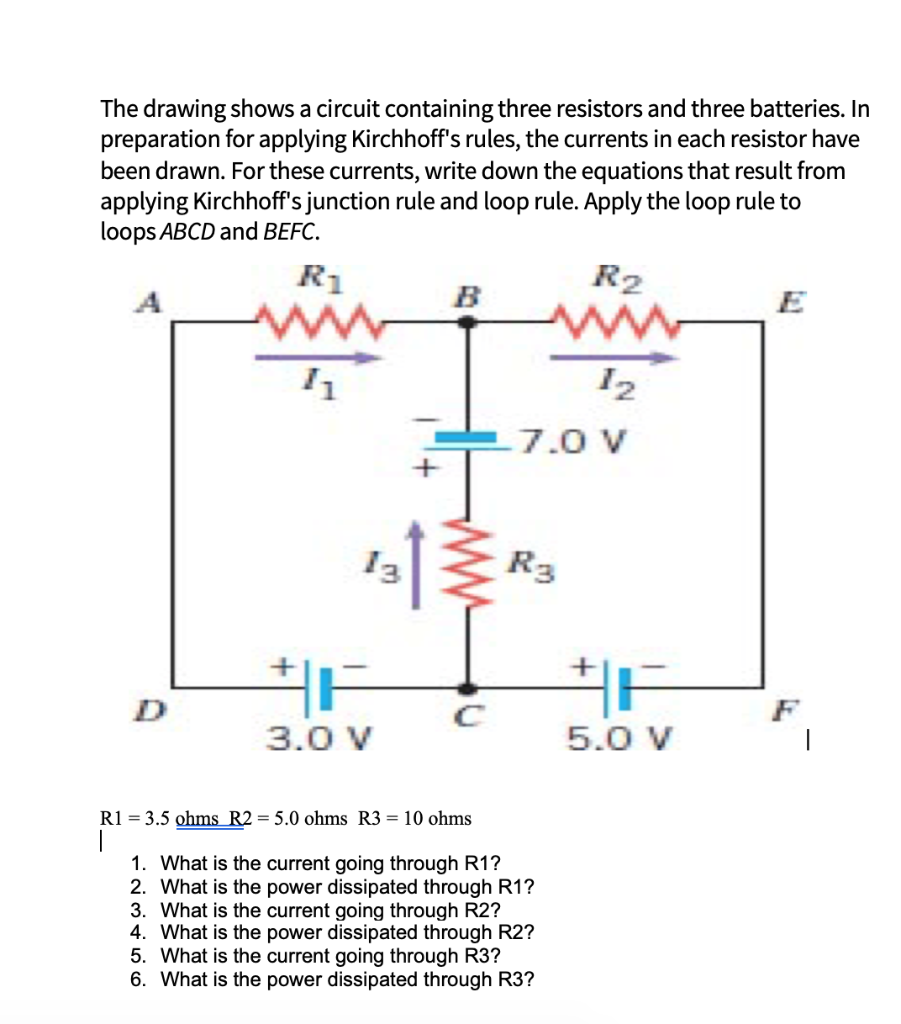
Solved The drawing shows a circuit containing three
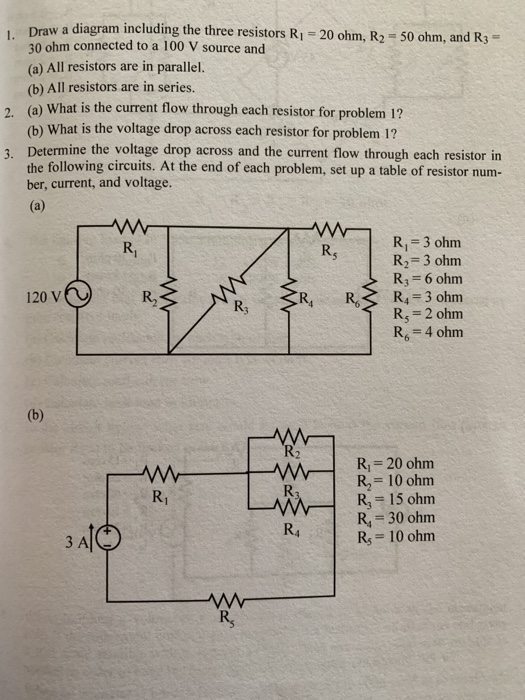
Solved Draw a diagram including the three resistors R1 = 20
Solved Each resistor in the three circuits in the drawing
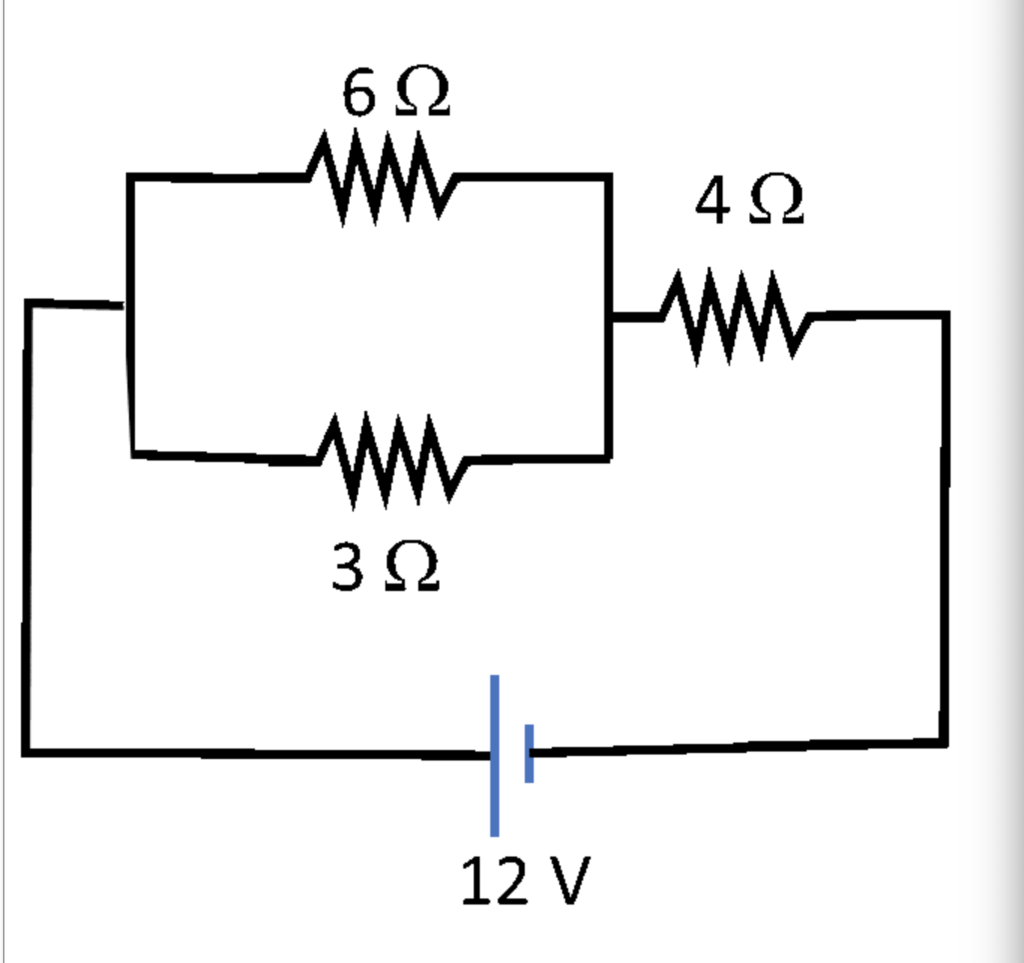
Solved Three resistors, R1=6 ohms, R2=3 ohms, and R3=5 ohms

Question 3 Each resistor in the three circuits in the drawing has the

Each resistor in the three circuits in the drawing has the same

Resistors Circuit Diagram
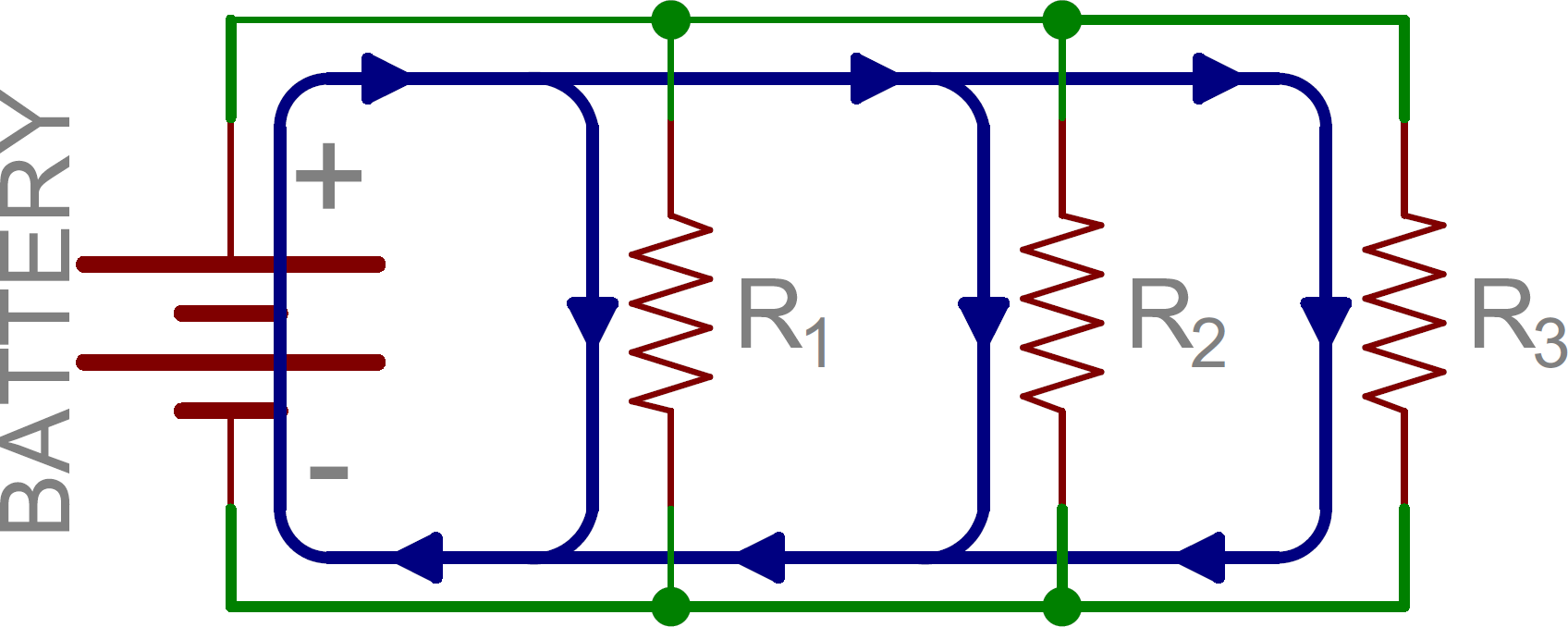
Series and Parallel Circuits SparkFun Learn

Resistors in Series and Parallel Electrical Academia
The Circuit In The Drawing Contains Three Identical Resistors.
Each Resistor In The Three Circuits In The Drawing Has The Same Resistance R, And The Batteries Have The Same Voltage V.
Determine The Total Power Delivered By The Battery In Each Of The Three Circuits.
The Current Through Each Resistor;
Related Post: